Discrete measure – Wikipedia

Posted on May 26, 2015 by lordneo

before-content-x4

From Wikipedia, the free encyclopedia

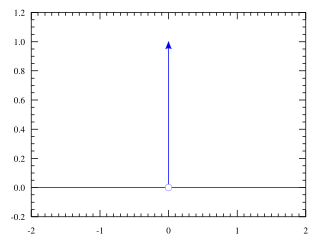
Schematic representation of the Dirac measure by a line surmounted by an arrow. The Dirac measure is a discrete measure whose support is the point 0. The Dirac measure of any set containing 0 is 1, and the measure of any set not containing 0 is 0.

after-content-x4

In mathematics, more precisely in measure theory, a measure on the real line is called a discrete measure (in respect to the Lebesgue measure) if it is concentrated on an at most countable set. The support need not be a discrete set. Geometrically, a discrete measure (on the real line, with respect to Lebesgue measure) is a collection of point masses.

Table of Contents

Definition and properties[edit]

A measure

{displaystyle mu }

$mu$ defined on the Lebesgue measurable sets of the real line with values in

after-content-x4

{displaystyle [0,infty ]}

$[0,infty ]$ is said to be discrete if there exists a (possibly finite) sequence of numbers

{displaystyle s_{1},s_{2},dots ,}

such that

{displaystyle mu (mathbb {R} backslash {s_{1},s_{2},dots })=0.}

The simplest example of a discrete measure on the real line is the Dirac delta function

{displaystyle delta .}

${displaystyle delta .}$ One has

{displaystyle delta (mathbb {R} backslash {0})=0}

${displaystyle delta (mathbb {R} backslash {0})=0}$ and

{displaystyle delta ({0})=1.}

${displaystyle delta ({0})=1.}$

More generally, if

{displaystyle s_{1},s_{2},dots }

$s_1, s_2, dots$ is a (possibly finite) sequence of real numbers,

{displaystyle a_{1},a_{2},dots }

$a_{1},a_{2},dots$ is a sequence of numbers in

{displaystyle [0,infty ]}

$[0,infty ]$ of the same length, one can consider the Dirac measures

{displaystyle delta _{s_{i}}}

${displaystyle delta _{s_{i}}}$ defined by

{displaystyle delta _{s_{i}}(X)={begin{cases}1&{mbox{ if }}s_{i}in X\0&{mbox{ if }}s_{i}not in X\end{cases}}}

for any Lebesgue measurable set

{displaystyle X.}

$X.$ Then, the measure

{displaystyle mu =sum _{i}a_{i}delta _{s_{i}}}

is a discrete measure. In fact, one may prove that any discrete measure on the real line has this form for appropriately chosen sequences

{displaystyle s_{1},s_{2},dots }

$s_1, s_2, dots$ and

{displaystyle a_{1},a_{2},dots }

$a_{1},a_{2},dots$

Extensions[edit]

One may extend the notion of discrete measures to more general measure spaces. Given a measurable space

{displaystyle (X,Sigma ),}

${displaystyle (X,Sigma ),}$ and two measures

{displaystyle mu }

$mu$ and

{displaystyle nu }

$nu$ on it,

{displaystyle mu }

$mu$ is said to be discrete in respect to

{displaystyle nu }

$nu$ if there exists an at most countable subset

{displaystyle S}

$S$ of

{displaystyle X}

$X$ such that

All singletons ${displaystyle {s}}$
${displaystyle nu (S)=0,}$
${displaystyle mu (Xbackslash S)=0.,}$

Notice that the first two requirements are always satisfied for an at most countable subset of the real line if

{displaystyle nu }

$nu$ is the Lebesgue measure, so they were not necessary in the first definition above.

As in the case of measures on the real line, a measure

{displaystyle mu }

$mu$ on

{displaystyle (X,Sigma )}

$(X,Sigma )$ is discrete in respect to another measure

{displaystyle nu }

$nu$ on the same space if and only if

{displaystyle mu }

$mu$ has the form

{displaystyle mu =sum _{i}a_{i}delta _{s_{i}}}

where

{displaystyle S={s_{1},s_{2},dots },}

${displaystyle S={s_{1},s_{2},dots },}$ the singletons

{displaystyle {s_{i}}}

${s_{i}}$ are in

{displaystyle Sigma ,}

$Sigma ,$ and their

{displaystyle nu }

$nu$ measure is 0.

One can also define the concept of discreteness for signed measures. Then, instead of conditions 2 and 3 above one should ask that

{displaystyle nu }

$nu$ be zero on all measurable subsets of

{displaystyle S}

$S$ and

{displaystyle mu }

$mu$ be zero on measurable subsets of

{displaystyle Xbackslash S.}

${displaystyle Xbackslash S.}$

References[edit]

Kurbatov, V. G. (1999). Functional differential operators and equations. Kluwer Academic Publishers. ISBN 0-7923-5624-1.

External links[edit]

after-content-x4

after-content-x4